European Spine Journal

September 2019, Volume 28, Issue 9, pp 1906–1913|

Hyun, SJ., Han, S., Kim, Y.B. et al. Eur Spine J (2019) 28: 1906. https://doi.org/10.1007/s00586-019-05955-w

Predictive formula of ideal lumbar lordosis and lower lumbar lordosis determined by individual pelvic incidence in asymptomatic elderly population

Abstract

Purpose

This study aimed to obtain reference values of lumbar lordosis (LL) and lower LL (LLL) from normal asymptomatic groups, determine the relationship between them and pelvic incidence (PI), and establish the predictive formula for ideal LLL in the elderly Korean population.

Methods

Standing plain lateral radiographs of the whole spine, including the pelvis, were analyzed in asymptomatic adult male volunteers without back pain or prior surgery involving the spine or lower extremity. Volunteers with scoliosis, spondylolisthesis, segmental disk space narrowing, and/or compression fractures in the radiographs were excluded. The following parameters were measured: LL, LLL, and pelvic parameters including PI. The values of PI–LL and PI–LLL were calculated, and the formula using stepwise multiple regression analysis was made. A P value of < 0.05 was considered statistically significant.

Results

The study participants included 150 volunteers. All were male participants with an average age of 64.1 ± 6.4. The average value of height, weight, and body mass index was 167.0 ± 5.5 cm, 67.3 ± 9.8 kg, and 24.1 ± 3.1 kg/m², respectively. The average LL was − 57.5° ± 9.0°, LLL was − 41.7° ± 7.0°, and PI was 48.6° ± 8.6°. The formula was established as follows: PI–LL = 0.38 × PI − 27.61 (R² = 0.172), PI–LLL = 0.77 × PI − 28.69 (R² = 0.516), ideal LL = 0.62 × PI + 27.61 (R² = 0.348), and ideal LLL = 0.225 × PI + 28.63 (R² = 0.083).

Conclusions

The ideal values of PI–LL and PI–LLL were inconsistent, and they have a positive correlation with PI in asymptomatic elderly population. Our data would be helpful as a normal reference value of ideal LL and LLL according to PI.

Graphical abstract

These slides can be retrieved under Electronic Supplementary Material.

Keywords

Lumbar Lower lumbar Lordosis Pelvic incidence Elderly population 

Seung-Jae Hyun and Sanghyun Han have contributed equally to this work and co-first author.

Electronic supplementary material

The online version of this article ( https://doi.org/10.1007/s00586-019-05955-w) contains supplementary material, which is available to authorized users.

Introduction

Pelvic incidence (PI) is an individual anatomical parameter, and it does not change along lifetime in any condition [1, 2]. In pathological condition, although all the positional parameters may vary, only the PI can be used to predict the ideal sagittal shape of spine postoperatively. Therefore, attempts were made to explain pelvic and spinal sagittal parameters with PI [1, 2, 3, 4, 5].

Among these attempts, the formula “pelvic incidence minus lumbar lordosis (PI–LL)” has been used as the most useful analogue to present the lordosis angle of the lumbar spine when performing spinal reconstructive surgery [3, 5]. Although this formula is relatively reliable and simple to use, several difficulties are encountered when applying to specific areas of the spinal disease and treatment. First, this formula was derived from the health-related quality of life (HRQOL) of adult spinal deformity patients, not from those with a normal spine. Second, this formula applies to all adult patients diagnosed with spinal deformity and age over 18 years, not to the age range of the patients we want to treat. Thus, due to this broad indication, it can be assumed that this formula (PI–LL) has a wide range of values from − 9° to + 9°. Third, this formula computes only the entire lumbar lordosis, not the segmental angle in the lumbar spine. Recently, Inami et al. [6] reported that an optimal PI–LL value is not a constant value, and a significant correlation was shown between the PI–LL and PI.

Especially, lower lumbar spine surgeries comprise the majority of spinal surgeries performed by a spine surgeon. Barrey et al. [7] reported that two-thirds of the LL was located in lower lumbar spine between L4 and S1. With the advent in technologies, the precise lordosis if it is known with preoperative planning can be introduced. Therefore, knowing the normal value of LL and lower lumbar lordosis (LLL) according to the PI value is considered to be an essential step in setting goals for the treatment of degenerative spinal diseases. However, the precise value of lower lumbar lordosis and the relationship with PI have not been presented in normal elderly population. This study aimed to obtain reference values of LL and LLL from normal asymptomatic groups and determine the relationship between them and PI in the elderly Korean population. Moreover, the parameters obtained from this study if considered standard can be used to determine abnormal sagittal balance in pathological conditions.

Materials and methods

Patient population

This study was approved by an institutional review board. Sagittal spinal radiographic parameters between March 2007 and September 2010 from male volunteers who had a healthy asymptomatic spine were investigated. These volunteers were enrolled when they did not have any problems as follows: no history of trauma, spinal disease including scoliosis, spondylolisthesis, segmental disk space narrowing and compression fractures in standing plain lateral radiographs, history of spinal surgery, history of hip joint osteoarthritis or pain in the spine, hip and lower extremity. The subjects with an acute or chronic pain in the spine, hip and lower extremity were excluded.

Radiographic measurement

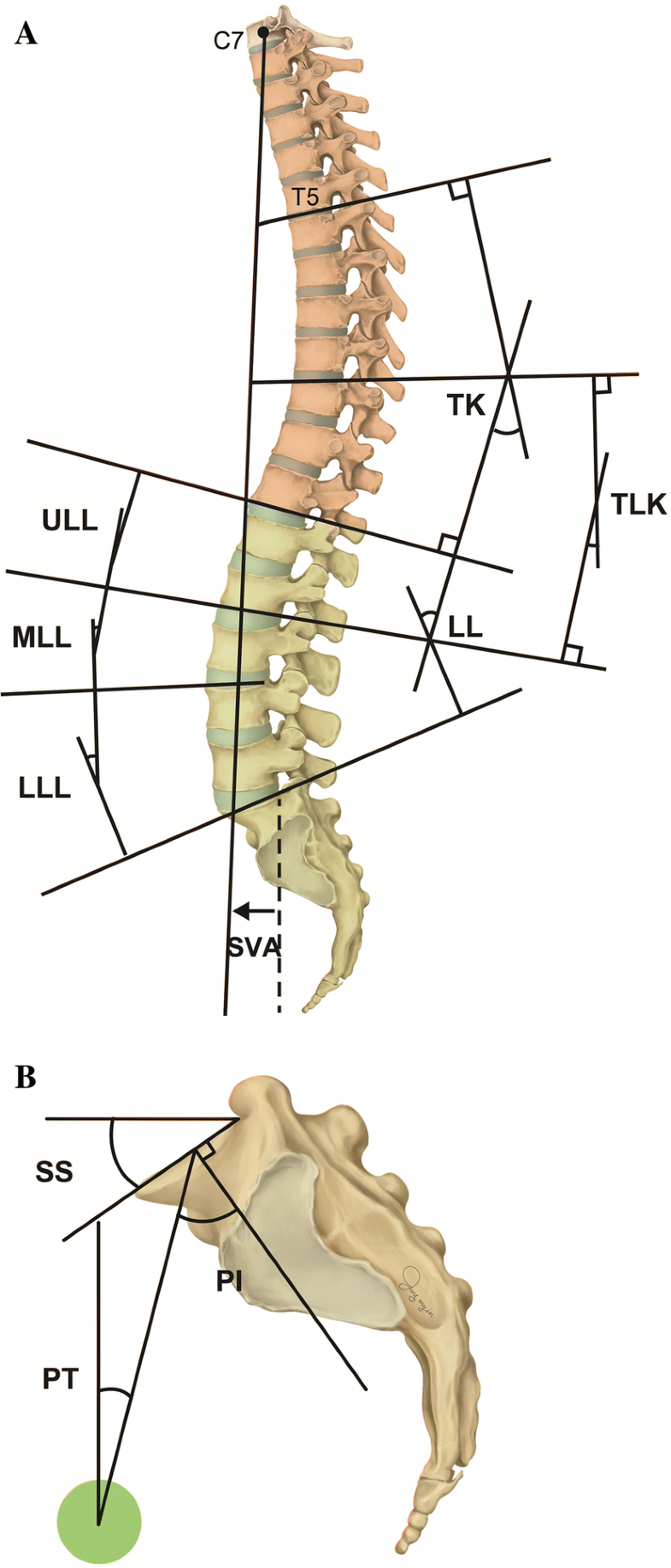
Standing plain lateral radiographs of the whole spine including the pelvis in asymptomatic adult male volunteers were analyzed. A total of 183 patients were recruited in this study, and the 33 subjects were excluded: There were seven cases of scoliosis over 10° in coronal plane, one case of pelvic tilting due to leg length discrepancy, eight cases of wedging of vertebra, nine cases with one or two segments narrowing, four cases of bilateral isthmic defect, and four cases of subjects with metallic foreign bodies (oriental acupuncture) in their back muscles. Finally, 150 patients were included in this study. The method of taking the standing radiographs was followed by that of Horton et al. [8]. Radiographs were stored into picture archiving and communication system (PACS, Maroview^®; Marotch, Seoul, Korea) in the format of digital imaging and communications in medicine (DICOM). Radiographic measurements were performed using a ruler and protractor function in Maroview^® PACS. Various sagittal distance and angular parameters were measured by a board-certified orthopedic spine surgeon (Y.B.K.).The distance parameters of sagittal vertical axis were measured plumb lines of C7 (C7PL), T12 (T12PL), and lumbar apex (LAPL) and that of the posterosuperior corner of S1 upper endplate (UEP). Sagittal angular parameters were as follows: thoracic kyphosis (TK) between T5 UEP and T12 lower endplate (LEP), thoracolumbar kyphosis (TLK) between T10 UEP and L2 LEP, and lumbar lordosis (LL) between T12 LEP and S1 UEP (negative value: lordosis; positive value: kyphosis). The LL was subdivided into three segments: upper lumbar lordosis (ULL) between T12 LEP and L2 LEP, middle lumbar lordosis (MLL) between L2 LEP and L4 UEP, and lower LL (LLL) between L4 UEP and S1 UEP, of which each has two disk spaces (Fig. 1a). Sagittal angular parameters in the pelvis were defined as follows: Sacral slope (SS) was defined as the angle between the horizontal line and the line composing S1 UEP, and pelvic incidence (PI) was the angle between the line perpendicular to the line composing S1 UEP at its center and the line connecting this point to the bicoxofemoral axis. Pelvic tilt (PT) was defined as the angle between the line connecting this center of S1 UEP to the bicoxofemoral axis and vertical line (Fig. 1b).

Statistical analysis

To quantify consistency of the results, we repeated the measurements of all the data. Intraclass correlation coefficients were calculated to determine how well the measurements were correlated (perfect reliability, 1.0; near-perfect reliability, 0.8–0.99; substantial reliability, 0.6–0.79; poor reliability, < 0.59). The correlations among various sagittal parameters were investigated using the Pearson correlation coefficient. The values from PI minus LL and PI minus each subpart of LL were calculated. The equations using simple linear regression analysis were made from the correlations between PI and PI–LL, PI–LLL, LL, or LLL. Then, predictive formula with fewer relevant variables including PI, the individual anatomical parameter, for LL and LLL was made using stepwise multiple regression analysis of the above parameters. A P value of < 0.05 was considered statistically significant. These statistical analyses were performed using the SPSS version 22.0 (IBM Corporation, Armonk, NY, USA).

Results

Intraclass correlation coefficient data and demographic data

All parameters of intraclass correlation coefficient are more than 0.8 except the lumbar apex (method of Shrout and Fleiss [9]), and they showed that they were near-perfect reliability between two measurements (Table 1). The study participants included 150 volunteers. All were male participants with an average age of 64.1 ± 6.4. The average value of height, weight, and body mass index (BMI) was 167.0 ± 5.5 cm, 67.3 ± 9.8 kg, and 24.1 ± 3.1 kg/m², respectively (Table 2).Table 1

ICC data of this study

ICC intraclass correlation coefficient, CI confidence intervalTable 2

Demographic data

SD standard deviation, No. number, BMI body mass index

Sagittal parameters

The distribution of volunteers according to the PI was as follows: PI < 40°, 40° ≤ PI < 50°, 50° ≤ PI < 60°, and 60° ≤ PI were 25, 68, 45, and 12, respectively. The sagittal parameters in this study were as follows: The distances from the posterosuperior corner of the sacral upper endplate to C7PL, T12PL, and LAPL were − 0.2 ± 2.8 cm, − 2.0 ± 1.7 cm, and 2.4 ± 0.7 cm, respectively. The sagittal spinal angular parameters were 30.0° ± 8.8° in TK, 9.9° ± 7.5° in TLK, and − 57.5° ± 9.0° in LL. In the lumbar lordosis, ULL, MLL, and LLL were − 1.9° ± 6.1°, − 16.1° ± 5.2°, and − 39.7° ± 6.8°, respectively. The pelvic parameters were 48.6° ± 8.6° in PI, 37.3° ± 6.8° in SS, and 11.3° ± 6.4° in PT (Table 3). The proportion of LLL against the LL was 69.7 ± 11.7%.Table 3

Sagittal parameters

SD standard deviation

Correlations among various sagittal spinopelvic parameters

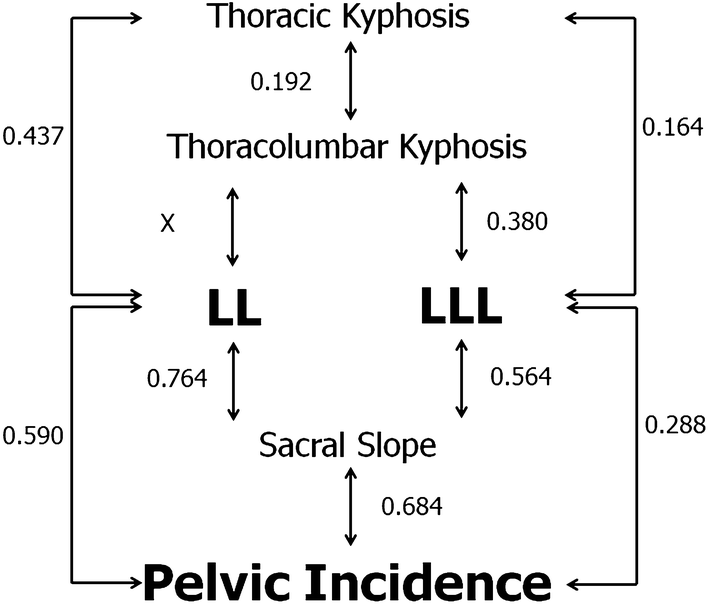
Strong correlations were observed between SS and LL (r = 0.764, P < 0.001) and SS and PI (r = 0.684, P < 0.001). PI was also correlated with LL (r = 0.590, P < 0.001) and LLL (r = 0.288, P < 0.001). There were correlations between LL and TK (r = 0.437, P < 0.001), LLL and TK (r = 0.164, P < 0.001), and LLL and sacral slope (r = 0.564, P < 0.001). These correlations are detailed in Fig. 2. Moreover, the PI is correlated with PI–LL (r = 0.415, P < 0.001) and PI–LLL (r = 0.718, P < 0.001).

Predictive formula of PI–LL, PI–LLL, LL, and LLL

The values of PI minus LL and PI–LLL were − 8.9° ± 7.7° and 9.0° ± 9.3°, respectively. The relationships between those values and PI were as follows. PI minus LL: PI–LL = 0.38 × PI − 27.61 (R² = 0.172); PI–LLL = 0.77 × PI − 28.69 (R² = 0.516) (Fig. 3).

The predictive formulas of LL and LLL were also established using those parameters with stepwise regression analysis. The following formula was established, and PI and TK are two important predictive variables for the lumbar lordosis: LL = 0.481 × PI + 0.295 × TK + 25.31 (R² = 0.428). PI and TLK are important factors for lower lumbar lordosis and formula as follows: LLL = 0.288 × PI + 0.338 × TLK + 21.36 (R² = 0.157).

Additionally, the ideal values for LL an LLL were obtained from simple linear regression analysis with PI. Ideal LL = 0.62 × PI + 27.61 (R² = 0.348, P < 0.001). Ideal LLL = 0.23 × PI + 28.63 (R² = 0.083, P < 0.001).

Discussion

Correlations among various sagittal spinopelvic parameters

In terms of anatomy, the relationship between LL and SS is essential. The larger the SS, the greater the value of LL. In addition, the thoracic spine and lumbar spine are connected through the thoracolumbar junction, and the curvature of each other is compensated for the uprightness of the human being. By the formula PI = SS + PT [1], the range of the SS is a value that cannot but be determined by PI. Therefore, the larger the PI, the greater the SS. Finally, because PI is the value of individual intrinsic value, we try to explain all the sagittal and pelvic parameters with PI. If so, LLL is a fraction of LL. Due to this point, the correlation coefficient between LLL and SS is smaller than the correlation coefficient of LL. Conversely, the fact that the correlation coefficients between LLL and SS are smaller than the values generated by LL and SS can be said to be the default values without large fluctuation. In other words, it can be said that the LLL basically does not change greatly according to the PI but must occupy a certain range of values.

Pelvic incidence and lumbar lordosis

It is of prime importance to make the balance in sagittal plane by the operation that creates the lumbar lordosis [10, 11, 12, 13]. Pelvic incidence plays a key role in the determination of the proper degree of lumbar lordosis in a patient [1, 4, 14, 15, 16, 17, 18]. Schwab et al. proposed a simple approach of “LL = PI ± 9°” based on the study of the relationship between lumbar lordosis and pelvic incidence using multiple regression analysis of an asymptomatic adult population (N = 48, mean age 48 ± 18), and it was derived from HRQOL score [2]. This formula has been verified by several authors [5, 19, 20, 21, 22, 23]. Nowadays, most reconstructive surgeries at the thoracic and lumbar spine have been aimed to meet the formula. The formula also offers the cutoff value within 10°, and a PI–LL mismatch is suggested as a potential risk factor for adjacent segment disease, proximal junctional kyphosis, and lumbopelvic fixation failure [22, 24, 25, 26]. A moderate correlation was obtained (R = 0.415, P < 0.001) with the correlation between PI–LL and PI. According to the result of this calculation, the explanatory power (R²) was 0.172. From our data, PI–LL is closely related with the PI. In other words, the value of PI–LL can be larger if the PI is larger or vice versa. This is in contrast to Schwab et al. [2] and Rothenfluh et al.’s [22] studies showing the limited range of normal PI–LL value that is desired to be corrected in pathological conditions. Knowing the characteristics of PI–LL in the asymptomatic population is necessary, and further research is needed to determine the HRQOL when this formula is set as the basis for treating patients with pathological conditions.

Lower lumbar lordosis

Although PI–LL criteria provided a valid indicator for determining the overall LL, it did not help to perform segmental fusion especially in the lower lumbar area or to perform entire lumbar fusion in harmonious shape [27]. The lower lumbar spine, defined as the upper endplate of the fourth lumbar vertebra to the sacrum, is the most important part of determining the lumbar lordosis [28, 29]. In addition, it is the most common area to do surgery in degenerative spinal diseases [30, 31, 32, 33]. Even if a single segment fixation is performed on the lower lumbar spine, the effect on the overall sagittal plane balance must be considered for optimal surgical planning. Therefore, we thought it would be important to see how LLL relates to the overall LL and other parameters. It is important to look at the relationship between LLL and other factors in the normal group and then to look at the relationship between the spine pathologic patients. Moreover, carrying out such research in the future will be necessary. In this study, we observed the correlations between PI and LLL, PI and LL (PI and LL [R = 0.590, P < 0.001] vs. PI and LLL [R = 0.288, P < 0.001]). Specifically, the correlations of PI–LLL and PI were significantly higher than that of PI–LL and PI (R² = 0.516 vs. R² = 0.172). Moreover, we also present the formulas of ideal LL and ideal LLL for PI in asymptomatic elderly population. To the best of our knowledge, no studies have investigated the ideal LLL according to PI in the asymptomatic elderly population. These results show that dividing the lumbar spine into segments rather than looking at the whole would be not only feasible but also helpful for surgical planning. However, because the formula is found in the asymptomatic population, further study of the relationship between PI–LLL and HRQOL is needed to find out whether this actually can be applied to surgical planning.

Age

Age is one of the important parameters affecting sagittal spinal parameters [34, 35, 36, 37]. A previous study presented the results from adults of a relatively wide age range (mean age 48 ± 18) [2]. When treating patients with degenerative changes, surgeons hope to obtain more accurate surgical target values according to the age of the patients than those presented by Schwab. We recruited healthy volunteers of the age-specific group (average age 64.1 ± 6.4) at which most degenerative changes in the spine develop well. These data can be a good reference for the treatment of degenerative spinal disease.

This study has several limitations. First, the subject is composed only of male individuals. We did not have any women volunteers because our hospital is for veterans. Second, we analyzed only subjects within a certain age range and could not see continuous sagittal changes according to all ages. However, most of the patients treated by spinal surgeons are of advanced age. We think that this study with old age group is so valuable for lumbar spine surgery and may also provide some directions for spinal surgery. Third, our study has adopted anatomical parameters rather than adopting geometric parameters. By doing this, patient with high pelvic incidence may put lot of lumbar lordosis in the thoracolumbar region, and if we just measure lumbar lordosis, there will be a high value of PI–LL though the patient is well balanced. However, adopting an anatomical measurement method rather than choosing a geometrical measurement may lead to a particular disagreement when making measurements. Moreover, the number of PI exceeding 60° is only about 8% of the total, and our predictive formula cannot be generalized in the people who have PI significantly exceeding 60°. However, the data concerning the number of patients with large PIs is small may be a characteristic of Korean elderly patients. Recent study showed similar results [38]. Finally, included people in this study were the current elderly patients in Korea and the fit BMI was a characteristic of the patients. However, it is difficult to apply our findings to higher BMI people. Future research involving people with evenly distributed BMI is also needed.

Conclusions

The ideal values of PI–LL and PI–LLL were inconsistent, and they have a positive correlation with PI in asymptomatic elderly population. Our data would be helpful as an important normal reference value of ideal LL and LLL according to PI.

Notes

Compliance with ethical standards

Conflict of interest

The authors declare that they have no conflict of interest.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary material

586_2019_5955_MOESM1_ESM.pptx (1.9 mb)Supplementary material 1 (PPTX 1941 kb)

References

1. 1.Boulay C, Tardieu C, Hecquet J, Benaim C, Mouilleseaux B, Marty C, Prat-Pradal D, Legaye J, Duval-Beaupere G, Pelissier J (2006) Sagittal alignment of spine and pelvis regulated by pelvic incidence: standard values and prediction of lordosis. Eur Spine J 15(4):415–422.  https://doi.org/10.1007/s00586-005-0984-5CrossRefGoogle Scholar
2. 2.Schwab F, Lafage V, Patel A, Farcy J (2009) Sagittal plane considerations and the pelvis in the adult patient. Spine (Phila Pa 1976) 34(17):1828–1833.  https://doi.org/10.1097/BRS.0b013e3181a13c08CrossRefGoogle Scholar
3. 3.Lafage V, Schwab F, Patel A, Hawkinson N, Farcy J (2009) Pelvic tilt and truncal inclination: two key radiographic parameters in the setting of adults with spinal deformity. Spine (Phila Pa 1976) 34(17):E599–E606.  https://doi.org/10.1097/BRS.0b013e3181aad219CrossRefGoogle Scholar
4. 4.Lafage V, Schwab F, Vira S, Patel A, Ungar B, Farcy JP (2011) Spino-pelvic parameters after surgery can be predicted: a preliminary formula and validation of standing alignment. Spine (Phila Pa 1976) 36(13):1037–1045.  https://doi.org/10.1097/brs.0b013e3181eb9469CrossRefGoogle Scholar
5. 5.Schwab F, Ungar B, Blondel B, Buchowski J, Coe J, Deinlein D, DeWald C, Mehdian H, Shaffrey C, Tribus C, Lafage V (2012) Scoliosis Research Society-Schwab adult spinal deformity classification: a validation study. Spine (Phila Pa 1976) 37(12):1077–1082.  https://doi.org/10.1097/brs.0b013e31823e15e2CrossRefGoogle Scholar
6. 6.Inami S, Moridaira H, Takeuchi D, Shiba Y, Nohara Y, Taneichi H (2016) Optimum pelvic incidence minus lumbar lordosis value can be determined by individual pelvic incidence. Eur Spine J. 25(1):3638–3643.  https://doi.org/10.1007/s00586-016-4563-8CrossRefGoogle Scholar
7. 7.Barrey C, Roussouly P, Le Huec J-C, D’Acunzi G, Perrin G (2013) Compensatory mechanisms contributing to keep the sagittal balance of the spine. Eur Spine J 22(6):834–841.  https://doi.org/10.1007/s00586-013-3030-zCrossRefGoogle Scholar
8. 8.Horton WC, Brown CW, Bridwell KH, Glassman SD, Suk S-I, Cha CW (2005) Is there an optimal patient stance for obtaining a lateral 36” radiograph?: a critical comparison of three techniques. Spine (Phila Pa 1976) 30(4):427–433CrossRefGoogle Scholar
9. 9.Shrout PE, Fleiss JL (1979) Intraclass correlations: us
   es in assessing rater reliability. Psychol Bull 86(2):420–428CrossRefGoogle Scholar
10. 10.Glassman SD, Bridwell K, Dimar JR, Horton W, Berven S, Schwab F (2005) The impact of positive sagittal balance in adult spinal deformity. Spine (Phila Pa 1976) 30(18):2024–2029CrossRefGoogle Scholar
11. 11.Kawakami M, Tamaki T, Ando M, Yamada H, Hashizume H, Yoshida M (2002) Lumbar sagittal balance influences the clinical outcome after decompression and posterolateral spinal fusion for degenerative lumbar spondylolisthesis. Spine (Phila Pa 1976) 27(1):59–64CrossRefGoogle Scholar
12. 12.Lazennec JY, Ramare S, Arafati N, Laudet CG, Gorin M, Roger B, Hansen S, Saillant G, Maurs L, Trabelsi R (2000) Sagittal alignment in lumbosacral fusion: relations between radiological parameters and pain. Eur Spine J 9(1):47–55CrossRefGoogle Scholar
13. 13.Liu H, Li S, Wang J, Wang T, Yang H, Li Z, Li X, Zheng Z (2014) An analysis of spinopelvic sagittal alignment after lumbar lordosis reconstruction for degenerative spinal diseases: how much balance can be obtained? Spine (Phila Pa 1976) 39(26):B52–B59.  https://doi.org/10.1097/brs.0000000000000500CrossRefGoogle Scholar
14. 14.Duval-Beaupere G, Schmidt C, Cosson P (1992) A Barycentremetric study of the sagittal shape of spine and pelvis: the conditions required for an economic standing position. Ann Biomed Eng 20(4):451–462CrossRefGoogle Scholar
15. 15.Legaye J, Duval-Beaupere G, Hecquet J, Marty C (1998) Pelvic incidence: a fundamental pelvic parameter for three-dimensional regulation of spinal sagittal curves. Eur Spine J 7(2):99–103CrossRefGoogle Scholar
16. 16.Kang KB, Ahn YJ, Kim YJ, Kim YB, Park SC (2011) Changes in sagittal spinopelvic parameters according to pelvic incidence in asymptomatic old Korean men. J Korean Soc Spine Surg 18(4):223–229.  https://doi.org/10.4184/jkss.2011.18.4.223CrossRefGoogle Scholar
17. 17.Tanguay F, Mac-Thiong JM, de Guise JA, Labelle H (2007) Relation between the sagittal pelvic and lumbar spine geometries following surgical correction of adolescent idiopathic scoliosis. Eur Spine J 16(4):531–536.  https://doi.org/10.1007/s00586-006-0238-1CrossRefGoogle Scholar
18. 18.Rose PS, Bridwell KH, Lenke LG, Cronen GA, Mulconrey DS, Buchowski JM, Kim YJ (2009) Role of pelvic incidence, thoracic kyphosis, and patient factors on sagittal plane correction following pedicle subtraction osteotomy. Spine (Phila Pa 1976) 34(8):785–791.  https://doi.org/10.1097/BRS.0b013e31819d0c86CrossRefGoogle Scholar
19. 19.Ha KY, Jang WH, Kim YH, Park DC (2016) Clinical relevance of the SRS-Schwab classification for degenerative lumbar scoliosis. Spine (Phila Pa 1976) 41(5) E282–288.  https://doi.org/10.1097/brs.0000000000001229CrossRefGoogle Scholar
20. 20.Sun XY, Zhang XN, Hai Y (2017) Optimum pelvic incidence minus lumbar lordosis value after operation for patients with adult degenerative scoliosis. The Spine J 17(7):983–989.  https://doi.org/10.1016/j.spinee.2017.03.008CrossRefGoogle Scholar
21. 21.Yamada K, Abe Y, Yanagibashi Y, Hyakumachi T, Satoh S (2015) Mid- and long-term clinical outcomes of corrective fusion surgery which did not achieve sufficient pelvic incidence minus lumbar lordosis value for adult spinal deformity. Scoliosis 10(Suppl 2):S17.  https://doi.org/10.1186/1748-7161-10-s2-s17CrossRefGoogle Scholar
22. 22.Rothenfluh DA, Mueller DA, Rothenfluh E, Min K (2015) Pelvic incidence-lumbar lordosis mismatch predisposes to adjacent segment disease after lumbar spinal fusion. Eur Spine J 24(6):1251–1258.  https://doi.org/10.1007/s00586-014-3454-0CrossRefGoogle Scholar
23. 23.Aoki Y, Nakajima A, Takahashi H, Sonobe M, Terajima F, Saito M, Takahashi K, Ohtori S, Watanabe A, Nakajima T, Takazawa M, Orita S, Eguchi Y, Nakagawa K (2015) Influence of pelvic incidence-lumbar lordosis mismatch on surgical outcomes of short-segment transforaminal lumbar interbody fusion. BMC Musculoskelet Disord 16:213.  https://doi.org/10.1186/s12891-015-0676-1CrossRefGoogle Scholar
24. 24.Cho W, Mason JR, Smith JS, Shimer AL, Wilson AS, Shaffrey CI, Shen FH, Novicoff WM, Fu KM, Heller JE, Arlet V (2013) Failure of lumbopelvic fixation after long construct fusions in patients with adult spinal deformity: clinical and radiographic risk factors. J Neurosurg Spine 19(4):445–453.  https://doi.org/10.3171/2013.6.spine121129CrossRefGoogle Scholar
25. 25.Hyun S-J, Rhim S-C (2010) Clinical outcomes and complications after pedicle subtraction osteotomy for fixed sagittal imbalance patients: a long-term follow-up data. J Korean Neurosurg Soc 47(2):95–101.  https://doi.org/10.3340/jkns.2010.47.2.95CrossRefGoogle Scholar
26. 26.Hyun SJ, Lee BH, Park JH, K
    im KJ, Jahng TA, Kim HJ (2017) Proximal junctional kyphosis and proximal junctional failure following adult spinal deformity surgery. Korean J Spine 14(4):126–132.  https://doi.org/10.14245/kjs.2017.14.4.126CrossRefGoogle Scholar
27. 27.Yilgor C, Sogunmez N, Boissiere L, Yavuz Y, Obeid I, Kleinstuck F, Perez-Grueso FJS, Acaroglu E, Haddad S, Mannion AF, Pellise F, Alanay A (2017) Global alignment and proportion (GAP) score: development and validation of a new method of analyzing spinopelvic alignment to predict mechanical complications after adult spinal deformity surgery. J Bone Joint Surg Am 99(19):1661–1672.  https://doi.org/10.2106/jbjs.16.01594CrossRefGoogle Scholar
28. 28.Roussouly P, Gollogly S, Berthonnaud E, Dimnet J (2005) Classification of the normal variation in the sagittal alignment of the human lumbar spine and pelvis in the standing position. Spine (Phila Pa 1976) 30(3):346–353CrossRefGoogle Scholar
29. 29.Sohn S, Chung CK, Kim YJ, Han I, Kang SM, Yoon JW, Kim H (2017) Sagittal spinal alignment in asymptomatic patients over 30 years old in the Korean population. Acta Neurochir 159:1119–1128.  https://doi.org/10.1007/s00701-017-3100-9CrossRefGoogle Scholar
30. 30.Cho JH, Joo YS, Lim C, Hwang CJ, Lee DH, Lee CS (2017) Effect of one- or two-level posterior lumbar interbody fusion on global sagittal balance. The Spine J 17(12)1794–1802.  https://doi.org/10.1016/j.spinee.2017.05.029CrossRefGoogle Scholar
31. 31.Kim MK, Lee SH, Kim ES, Eoh W, Chung SS, Lee CS (2011) The impact of sagittal balance on clinical results after posterior interbody fusion for patients with degenerative spondylolisthesis: a pilot study. BMC Musculoskelet Disord 12:69.  https://doi.org/10.1186/1471-2474-12-69CrossRefGoogle Scholar
32. 32.Lee CH, Chung CK, Jang JS, Kim SM, Chin DK, Lee JK, Yoon SH, Hong JT, Ha Y, Kim CH, Hyun SJ (2017) Effectiveness of deformity-correction surgery for primary degenerative sagittal imbalance: a meta-analysis. J Neurosurg Spine 27(5):540–551.  https://doi.org/10.3171/2017.3.spine161134CrossRefGoogle Scholar
33. 33.Han S-H, Hyun S-J, Jahng T-A, Kim K-J (2015) A comparative radiographic analysis of fusion rate between L4-5 and L5-S1 in a single level posterior lumbar interbody fusion. Korean J Spine 12(2):60–67CrossRefGoogle Scholar
34. 34.Hasegawa K, Okamoto M, Hatsushikano S, Shimoda
    H, Ono M, Watanabe K (2016) Normative values of spino-pelvic sagittal alignment, balance, age, and health-related quality of life in a cohort of healthy adult subjects. Eur Spine J 25(11):3675–3686.  https://doi.org/10.1007/s00586-016-4702-2CrossRefGoogle Scholar
35. 35.Kang KB, Kim YJ, Muzaffar N, Yang JH, Kim YB, Yeo ED (2010) Changes of sagittal spinopelvic parameters in normal Koreans with age over 50. Asian Spine J 4(2):96–101.  https://doi.org/10.4184/asj.2010.4.2.96CrossRefGoogle Scholar
36. 36.Mac-Thiong JM, Roussouly P, Berthonnaud E, Guigui P (2011) Age-and sex-related variations in sagittal sacropelvic morphology and balance in asymptomatic adults. Eur Spine J 20(Supp 5):572–577.  https://doi.org/10.1007/s00586-011-1923-2CrossRefGoogle Scholar
37. 37.Sasaki K, Hongo M, Miyakoshi N, Matsunaga T, Yamada S, Kijima H, Shimada Y (2017) Evaluation of sagittal spine-pelvis-lower limb alignment in elderly women with pelvic retroversion while standing and walking using a three-dimensional musculoskeletal model. Asian Spine J 11(4):562–569.  https://doi.org/10.4184/asj.2017.11.4.562CrossRefGoogle Scholar
38. 38.Tono O, Hasegawa K, Okamoto M, Hatsushikano S, Shimoda H, Watanabe K, Harimaya K (2018) Lumbar lordosis does not correlate with pelvic incidence in the cases with the lordosis apex located at L3 or above. Eur Spine J.   https://doi.org/10.1007/s00586-018-5695-9 [Epub ahead of print]Google Scholar

Copyright information

© The Author(s) 2019

Open AccessThis article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.